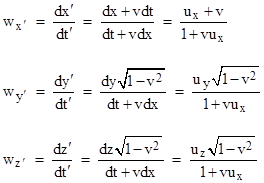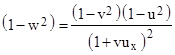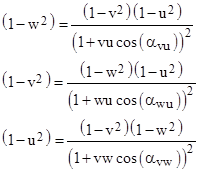Velocity Compositions and Rapidity

The sole purpose of this little paper was to show that Minkowski's profound conception of spacetime not only facilitates a systematic understanding of the general structure of the relativity theory, but also serves as a convenient guide for specific questions.

                                                                                                                Sommerfeld, 1909

Consider two particles at the origin of inertial coordinates x,y,z,t, and suppose that, in terms of these coordinates, the first particle is moving with velocity –v and the second is moving with velocity u. Also, let w denote the velocity of the second particle in terms of the rest frame coordinates of the first particle. Since the origin of the x,y,z,t coordinates is moving with velocity v relative to the rest frame coordinates of the first particle, and the second particle is moving with velocity u relative to the x,y,z,t coordinates, we say that w is the composition of v and u. Notice that we can always perform a spatial rotation of the original coordinates so that v is in the negative x direction, so there is no loss of generality in stipulating that the first particle is moving at the speed v in the negative x direction.

Letting t′,x′,y′,z′ denote rest frame coordinates for the first particle (suitably aligned so that the x’ and x axes coincide, etc.), we know that these are related to the original coordinates by the standard Lorentz transformation for speed v in the negative x direction, which we can write in differential form as

An incremental segment dx,dy,dz of the worldline of the second particle has velocity components u_x = dx/dt, u_y = dy/dt, and u_z = dz/dt, so it follows that the components of the velocity of this worldline in terms of the primed coordinates (i.e., the rest frame coordinates of the first particle) are

Note that if the velocities u and v are co-linear, meaning we have u_x = u and u_y = u_z = 0, then this reduces to the familiar one-dimensional speed composition formula w = (u+v)/(1+uv). In general, the magnitude w of the vector w is given by

Substituting for the components on the right side, and re-arranging terms, this gives the relation

Letting α denote the angle (in terms of the original x,y,z,t axes) between u and v (the latter coinciding with the x axis), we have u_x = u cos(α), so this relation can be written as

In this form the relation clearly applies to any two velocity vectors, not just to cases when v is along the x axis. If we take the reciprocal square roots of both sides, this is equivalent to the expression

This expression suggests that with any given speed v we associate an angle ϕ_v, sometimes called the rapidity, defined by tanh(ϕ_v) = v. Since tanh = sinh/cosh, and cosh² – sinh² = 1, it follows that

Therefore in terms of the rapidities we can express the general formula for relativistic composition of velocities as

If u and v are colinear, and α = 0, and hence cos(α) = 1 and the right hand expression reduces to the trigonometric identity for the sum of ϕ_u and ϕ_v, so in that special case the rapidities are simply additive, i.e., we have ϕ_w = ϕ_v + ϕ_u. In the general case, when u and v are not co-linear, the rapidities are not simply additive, but they remain additive in a different sense.

To visualize the general case, it’s useful to consider a slightly different definition of rapidity. The definition given above was based on the hyperbolic tangent function, so we might call it the hyperbolic rapidity, but we could just as well have defined a spherical rapidity in terms of the circular tangent function by tan(φ_v) = iv. Since tan = sin/cos, and sin² + cos² = 1, it follows that

Therefore in terms of the spherical rapidities we can express the general formula for relativistic composition of velocities as

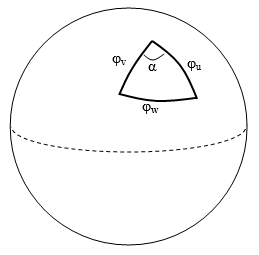
We recognize this from spherical trigonometry as the formula relating the edges (i.e., central arc lengths) of a geodesic triangle on a sphere of unit radius, as depicted below.

Note that the sign in the expression is reversed by changing the sign of φ_u relative to the sign of φ_v. The sign can also be reversed by using the complementary angle α between the two vectors. Letting α_v denote the interior angle opposite the rapidity φ_v, and so on, the angles between the rapidity arcs are related to the rapidity arc lenths themselves by the usual “law of sines”

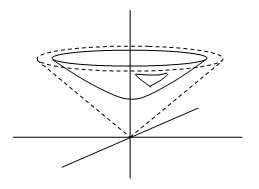
Of course, this correspondence between velocities and spherical arc lengths and angles is only formal, because it involves imaginary lengths and angles. In contrast, the hyperbolic rapidities defined previously correspond to real-valued angles, and the composition formula represents the “law of (hyperbolic) cosines” for “triangles” on a unit hyperboloid surface, as depicted below.

In this model the “line” between two points in the hyperboloid is the intersection between the hyperboloid and the plane containing those two points and the origin, and arc lengths are evaluated by integrating the Minkowski line element.

Returning to the velocity composition formula (1) in terms of velocities, notice that by symmetry we actually have the three relations

The angles in these formulas are each defined in terms of a different system of coordinates. The original angle α_vu (which we previously called simply α) is defined in terms of the rest frame of the origin of the x,y,z,t coordinates, whereas the angle α_vw is defined in terms of the rest frame of the first particle, and the angle α_uw is defined in terms of the rest frame of the second particle. Unlike the angles of a triangle on a flat Euclidean plane, these three angles do not sum to π. As a result, the composition of two given vectors is not commutative, which leads to phenomena such as Thomas precession.

Return to MathPages Main Menu