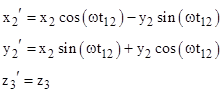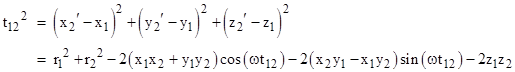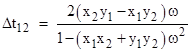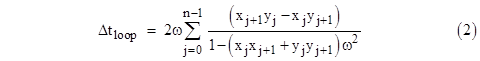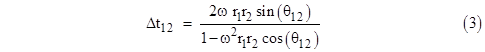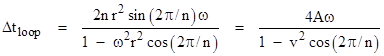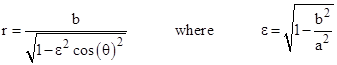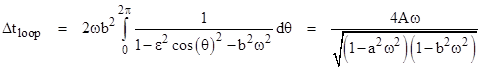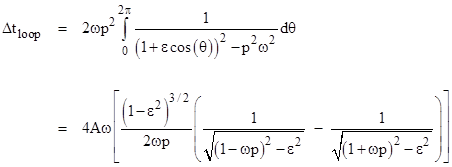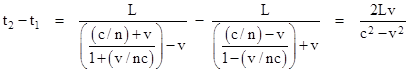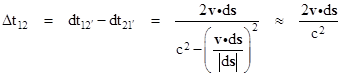Sagnac and Fizeau

A derivation of the Sagnac effect for a set of mirrors arranged at the vertices of a regular polygon rotating about its center was presented in a previous article. There we considered only signals moving at the speed of light in vacuum. Here we present a derivation for arbitrary arrangements of mirrors rotating about any point, and we show how this gives an exact solution for arbitrary continuous paths (such as through optical fibers) with arbitrary index of refraction. This analysis is also shown to be applicable to signals of any kind, provided only that they move isotropically with respect to inertial coordinates instantaneously co-moving with the local rotating medium. Lastly, we show that the same results apply to a simpler class of arrangements, in which the light follows a fixed closed loop in both directions, and the optical medium moves everywhere tangential to the path, as would be the case with a flexible loop of optical fiber moving like a conveyor belt.

First we consider an arbitrary planar configuration of mirrors rotating about a single point. For an inertial coordinate system x,y,z,t in terms of which the center of rotation is at rest at the origin, let x₁,y₁,z₁ and x₂,y₂,z₂ denote the coordinates of two consecutive mirrors on the path at the time t = 0. Also, let r₁ and r₂ denote the distances of these two points from the origin. Without loss of generality we can assume the configuration of mirrors is rotating in unison with an angular speed ω about the z axis. At the time t = 0 a pulse of light is emitted from the first mirror and arrives at the second mirror after an elapsed time of t₁₂. During this time the second mirror has moved to the point

Considering first the case of light signals in vacuum, we know the speed of light is 1 (with suitable units), so we have

Expanding the cosine and sine functions up to second order in ωt₁₂ (assuming this angle, which represents the amount of rotation of the mirrors during the transit of the signal from one mirror to the next, is very small compared to 1) and re-arranging terms gives the quadratic

where L₁₂ is the distance from point 1 to point 2. The analogous quadratic for a light pulse going from point 2 to point 1 is identical except with the opposite sign for the second term. The two roots of these polynomials are the two time intervals with opposite signs, so the sum of the roots of either quadratic gives the difference between the times. Therefore, the difference in times for the segment between the mirrors at points 1 and 2 is

So, given a closed loop of n mirror positions x_j,y_j for j = 0 to n-1, the total time difference for two pulses of light to traverse the loop in opposite directions can be computed by the summation

with the understanding that the indices are reduced modulo n. (Note that variations in the z coordinates do not affect this time difference.) We recognize the sum of the numerators as simply twice the net  area within the loop, and the denominators are very nearly equal to 1, provided the circumferential speed of the loop is small compared to c. This explains why, to the lowest non-zero order, the difference between the transit times around the loop in opposite directions is simply 4Aω, which is 4Aω/c² in arbitrary units. This applies to any arrangement of mirrors, all rotating in unison about any point.

Noting the trigonometric identities

where θ₁₂ is the angle between the vectors r₁ and r₂, we can also write the time difference per segment in the form

As an example, consider a regular n-sided polygon centered on the axis of rotation, with n vertices at a distance r from the axis. In this case the total time difference for all n segments around the loop is

where A is the total area of the n-gon and v is the circumferential speed of the vertices. In the limit as n goes to infinity we have the time delay for a circular loop

The preceding analysis was based on light signals propagating through a vacuum, so the elapsed proper time for the light pulse was null in both directions. Actual circular loops are often constructed using continuous fiber optic wire, which has an index of refraction, n, significantly different from 1. In this case the pulses of light will not propagate at the speed c but at the speed u = c/n relative to the medium, and therefore the transit intervals will not be null. In fact, we need not restrict ourselves to light signals. We can consider any signal propagating at the speed u (less than or equal to c) relative to inertial coordinates instantaneously co-moving with the local medium. If u is less than c, the elapsed proper time for traversing an incremental segment of a continuous spatial path through the medium is non-zero, but just as in the case when u = c it is the same in both directions (due to isotropy of the co-moving inertial coordinates).

Therefore, given either a continuous path or else a path with segments small enough to be treated as inertial over the time period required for the signal to traverse from one mirror to the next, equation (1) still applies, except that instead of the left hand expression equaling zero, it equals some non-zero (dτ)². Thus we have, for an incremental segment from point 1 to point 2 along the path

Again the equations of transit in the opposite direction are identical except for the sign of the second term, so the difference between the times t₁₂ and t₂₁ is again given by the sum of the roots of either of these equations. Thus for an incremental portion of the path the difference in coordinate times for the transit in the two directions has the form of equation (3), and in the continuous limit as θ₁₂ goes to zero we have the differential

This applies regardless of the speed u of the signal, provided only that u is the speed of the signal relative to inertial coordinates instantaneously co-moving with the medium. For example, if two men carry ideal clocks in opposite directions around a closed loop path (not necessarily circular) drawn on a rotating platform, and they each maintain a fixed speed relative to the portion of the platform where they are located at any given instant, then the elapsed times on their respective clocks required to go completely around the loop will be identical. However, their arrival times will be different, by the amount given by integrating equation (5) around the loop. Thus for any continuous spatial closed loop path and any signal speed, the time difference is

Notice that, in general

where A is the net area of the loop, so the integral of just the numerator of (6) around the loop would be 4Aω. The denominator of equation (1) is 1 – v² where v is the circumferential speed of the respective point of the loop.

Equation (6) is exact for any continuous path that is stationary with respect to a particular system of uniformly rotating coordinates. We might be tempted to try to use this to give an exact solution for a polygonal arrangement of discrete mirrors, assuming the path follows the straight line between the mirrors, but this would not be valid, because the signals moving in opposite directions do not trace the same paths between discrete mirrors. In terms of a system of coordinates in which the mirrors are stationary, the co-rotating signal passes slightly inside the stationary straight line between two mirrors, and the counter-rotating signal passes slight outside. Hence equation (6) is exact only for continuously specified paths, such as are given by an optical fiber (or people walking along a continuous path painted on a rotating platform).

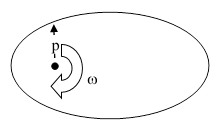
To illustrate, consider an elliptical loop of fiber optic wire, rotating at constant angular speed ω about its center, as shown below.

The points of the path are specified by

Inserting this into equation (6) and evaluating the integral gives

where A = abπ is the area of the ellipse. If a = b = r we have a circular loop of radius r with v = ωr, and this equation reduces to (4) as required. For another example, suppose the elliptical loop of fiber optic wire is rotating about one foci, as shown below.

In this case the rotating spatial path is defined by

where p = b²/a, so the time difference is given by

For a circular loop of radius r we have ε = 0 and p = r, and this equation reduces to (4) as required. All three of these examples (a circular loop rotating about its center, an elliptical loop rotating about its center, and an elliptical loop rotating about one focus) give the same result, 4Aω/c, to the first order in v/c. They differ from each other only in the second order in v/c. For more example, see the note on Integrating Polygonal Sagnac Paths.

Incidentally, the question sometimes arises as to whether the Sagnac effect is “classical” or requires a relativistic explanation. The question is complicated by the fact that there were many different "classical" theories of optics. In ballistic theories (that are consistent with, e.g., Michelson-Morley) the speed of light is the sum of the speed of the source plus a velocity of magnitude c. On this basis, there should be no Sagnac effect at all (for a circular loop), so these theories can be ruled out. (This is true even for the somewhat contrived pseudo-emission theories such as that of Ritz, which postulates different behavior for light emitted from mirrors than from other materials.) On the other hand, some classical wave theories in which light propagates through an ether are nominally consistent with the Sagnac effect, at least in vacuum. If we construct a Sagnac device with some optical medium like glass fibers, the question becomes still more complicated, because within the class of ether wave theories there were many different ideas about how the "ether" interacted with ordinary matter, if at all. To account for all first-order optical effects, including the Fizeau experiment – which showed how light propagates through a moving column of water – classical theorists adopted Fresnel's "partial dragging" hypothesis. This meant the ether was neither totally stationary nor totally dragged along by material bodies. Through a complicated chain of classical reasoning, Fresnel actually predicted this partial dragging in 1818, three decades before Fizeau performed his experiment. According to Fresnel, the speed of light in a medium with refractive index n moving (along the same line) at speed ±v should be c/n ± v(1 – n²), and of course Fizeau confirmed this (up to first order). Using Fresnel’s hypothesis, we find that it predicts a Sagnac effect in agreement up to first order with the relativistic prediction, basically because Fresnel's partial dragging formula mimics the relativistic speed composition rule up to first order.

Of course, Fresnel's interpretation of the extra term in the velocity as being due to partial dragging of the ether is somewhat problematic in itself, because the index of refraction in material media varies with frequency, which means that Fresnel needs infinitely many ethers, one for each frequency of light, being dragged at slightly different speeds, to account for the observed behavior at all frequencies. This is the kind of detail that always nagged at ether theorists, but in general they were happy to just have a theory that more or less agreed with all the first-order experiments. It was the second order experiments that made it clear to everyone that the relativity principle itself, rather than the old mechanical principles, was the more reliable guide to how things work.

When Sagnac performed his experiment in 1913 most French physicists still had not taken notice of Einstein’s interpretation of Lorentz’s relativistic ether theory. For Sagnac, the two main competing theories of light were still the ether/wave theory of Lorentz and the ballistic corpuscle theory of people like Ritz. Those were the two main traditions, going back to Huygens and Newton respectively. One major distinction between these theories is that in an ether theory the speed of light is independent of the speed of the source, whereas in a ballistic theory the speed of the source is added to the speed of light. Sagnac's conclusion in his 1913 paper was that (in his words) "the speed of light is independent of the speed of the source". This, he declared, proves the existence of an ether. Needless to say, the independence of light speed from the speed of the source is a fundamental property of Einstein's relativity theory too, so no one but the supporters of ballistic theories was ever bothered by Sagnac's observations. Sagnac's paper didn't discuss the possibility of a non-ether theory with invariant light speed. He simply equated the source-independence of light speed with proof of an ether/wave theory.

There is a mythology among many modern crackpots that Sagnac's result was a refutation of special relativity, and that therefore the effect was for decades ignored by the scientific community. This mythology is both technically and historically untrue. First, as noted above, Sagnac’s conclusion was simply that the speed of light is independent of the source, a fact which is in perfect accord with special relativity. Second, when someone named Paul Harzer published a note in 1914 suggesting that the effect (referring to Harress’s work on light propagating in a rotating medium) was inconsistent with special relativity, Einstein immediately (July 1914) responded in the same journal, clearly explaining the fallacy in Harzer’s reasoning:

Mr. Harzer states that in accordance with relativity theory the convection coefficient (1c) is to be expected, while he finds from the experiment of Harress that the results are in accordance with convection coefficients (1b).  A view of the Harress arrangement shows however that it quite concerns the case (1b) here, so the experiment as well as Harzer’s calculation supplies not a refutation but, to the contrary, a confirmation of the theory.

Everyone familiar with special relativity, even critics such as Michelson, always recognized that the Sagnac effect is a (rather trivial) confirmation of special relativity, not a refutation.

Another part of the anti-relativityist mythology is the idea that the Michelson-Gale measurement of the Earth’s rotation in 1924 by means of the Sagnac effect refutes special relativity, and/or was viewed as such at the time. This again is utterly false, both technically (for the reason given above) and historically. The measurement was first considered by Michelson around 1905, but he realized it would not discriminate between the predictions of special relativity and those of a stationary ether theory (with no drag), so he did not pursue it. The idea was raised again, by a certain Dr. Ludwig Silberstein, in 1921 during Einstein’s visit to the United States. According to the rather breathless account given in the New York Times for May 12

A proposal for an experiment which may prove Einstein's theory of relativity to be all wrong has been placed before scientific men here to attend Professor Einstein's lectures, and it has aroused the greatest interest. This is to test the pull of the rotating earth upon the ether to learn whether there is a drag, whole or partial, and it has several possible results, the most important of which is its effect on the theory of relativity. So important is the experiment judged to be by those who have learned of it that Professor Albert A. Michelson… has offered to perform the experiment. Professor Einstein was informed of it three days ago, and at first was inclined to doubt that it would have any bearing on his theory, but, after thinking it over, has decided that it is a new and practical way of testing his theory, and has described it as "wonderful." … Based on the ether theory the effect should be either equal to the full value [of the Sagnac effect], if there is no dragging of the ether by the spinning earth, and no effect at all if there is a full drag. Finally there would be only a fraction of the full effect if there is a partial dragging of the ether by the spinning earth. If, therefore, the experiment which Professor Michelson will perform gives a full value of the shift, this will harmonize with the general relativity theory as well as with the ether theory, but if the effect is nil, or only a fraction of the full shift of 1.4 per square kilometer, it will be "a death blow to the relativity theory," although compatible with the ether theory, testifying simply to a partial drag… Professor Einstein … said he would gladly recognize a fractional shift as a blow to his theory, and at the same time enjoy the demonstration of the novel phenomena. However, both Dr. Silberstein and Professor Einstein believe that the full shift effect will be shown.

Needless to say, when the measurement was actually performed, the full shift was observed, as Silberstein, Einstein, and Michelson had all known it would be, thereby demonstrating yet another phenomenon consistent with relativity. It would be fascinating to know how these mundane facts, which plainly describe a confirmation of relativity theory, came to be adopted into the anti-relativityist folklore as a canonical refutation of relativity.

Of course, as was obvious to Michelson and Einstein all along, this measurement [which was performed in as near to vacuum as possible) does not discriminate between relativity and a perfectly un-dragged ether, so it is a rather trivial confirmation of special relativity. However, it is also possible to perform such a measurement in a medium with an index of refraction differing from 1. Indeed many ordinary Sagnac devices using fiber optic lines and therefore actually involve the Fizeau effect as well as the Sagnac effect, because they run light in opposite directions through a rotating medium with an index of refraction differing significantly from 1. In order to account for the results in this kind of device, an etherist needs to invoke, at the very least, Fresnel's partial dragging hypothesis (whereas he needs to deny any dragging at all to account for the full shift measured in vacuum). This makes the device a somewhat less trivial confirmation of special relativity, because the Fizeau effect is not trivial. This is seldom mentioned in discussions of the Sagnac effect, perhaps justifiably, because the "pure" Sagnac effect consists of the path dependence of the optical path length with respect to a rotating system, as distinct from the Fizeau effect of light propagating in a moving medium. Nevertheless, both of these effects are present in many real Sagnac devices.

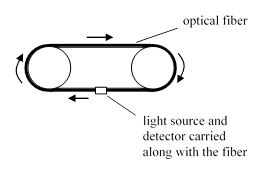
To conclude, we will consider the case of a flexible loop of optical fiber moving like a conveyor belt with some arbitrary shape. A simple example, with just two rollers, is depicted below.

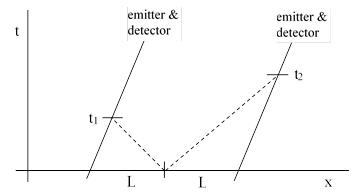
This is simpler than an ordinary Sagnac arrangement, because the “mirrors” all move directly along the path of the light, either toward or against the direction of the light. Thus it is essentially a one-dimensional problem, and the motions of the light pulses and the emitter/detector can be represented as shown below.

Letting n denote the index of refraction of the fiber, the speed of light relative to the fiber at any point is c/n, and the speed of light relative to the fixed inertial coordinates of the roller axes is given by the relativistic speed composition formula (c/n ± v)/(1 ± v/nc), so the difference between the arrival times t₁ and t₂ of pulses of light emitted from the source is

This applies to any shape of loop, provided the fiber always moves along the direction of the fiber at each point. Of course, for a circular loop, this setup is identical to a circular Sagnac device rotating about the center of the circle, in which case L equals the circumference 2πR of the loop, and v = ωR, so we have the usual formula 4Aω/(c² – v²) for the time difference, where A = πR² is the area of the loop. As with a Sagnac device, the belt loop time difference is independent of the index of refraction.

The belt loop arrangement is rather trivial, and might not be worth mentioning, although a couple of papers were published (in Phys Rev Let A) in 2003 and 2004 presenting results of measurements from such a device. Needless to say, the measured results agreed with the above formula, based on special relativity, for all shapes of the loop, and for all indices of refraction, exactly as one would expect. As to why such a trivial phenomenon deserved to be “tested”, the authors of those papers revealed (in another, less reputable, publication) that they believe it “falsifies the principle of the constancy of the velocity of light”, and therefore invalidates special relativity. Unfortunately, they don’t explain how experimental results that agree exactly with the predictions of special relativity can invalidate special relativity. This type of arrangement is essentially equivalent to having a combined light source and receiver moving between two parallel mirrors. Naturally the reflected light will be red-shifted from one mirror and blue-shifted from the other, depending on the state of motion of the source/receiver relative to the mirrors.

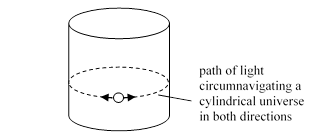
Of course, a closed topology for paths of light could also (conceivably) be achieved in other ways. One can imagine a cylindrical universe (for example), in which light is able to circumnavigate the closed dimension of the universe while always propagating in a straight line, as depicted in the figure below.

In this context there indeed is an absolute state of rest (in the cylindrical direction) that results in isotropic light in both directions, even though this state of rest is not defined relative to any substantial entity. This is not a new or novel realization. Symmetries such as Lorentz invariance and the isotropy of Euclidean space are essentially local. It is well known that asymmetries are introduced globally by defining some non-trivial global topology. For example, the surface of a cylinder is locally isotropic, but it obviously is not globally isotropic. Likewise Lorentz invariance applies locally, but not globally. This shows that Lorentzian relativity is even less of a relational theory than is Galilean relativity, because in a cylindrical universe under Galilean relativity (including a ballistic theory of light) there is no identifiable state of absolute rest, just as a pure ballistic theory predicts no Sagnac phase shift. The null cone structure of Minkowski spacetime entails a physically meaningful absolute rest (globally) when a closed cylindrical topology is imposed. Similar comments would apply to a closed spherical universe, or a torus, or any other non-trivial global topology that we might imagine. In all cases the spacetime is locally Lorentzian, so these consideration do not invalidate special relativity, which asserts only the local Lorentz invariance of physical phenomena, not global invariance. Of course, whether we regard this distinction as meaningful depends on whether we think it is plausible that the universe can be circumnavigated by causal effects. Also, we already know that global Lorentz invariance fails in the context of curved spacetime, such as in general relativity, where only local Lorentz invariance is maintained.

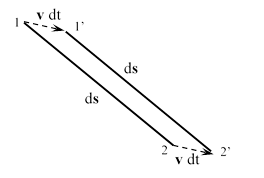
In both a Sagnac device and a fiber belt device there is only a single degree of freedom for the motions of the loop, and each pulse in a given direction travels a congruent path, modulo some offset. It’s easy to evaluate the more general case of arbitrary flexible motions of the fiber optic loop, provided the accelerations of various parts of the loop are sufficiently low, i.e., low enough so that the changes in velocities of the parts of the loop are negligible during the time required for a light pulse to travel around the loop. On this basis, we can consider the displacement of an arbitrarily short increment ds of the fiber loop as shown below.

If this segment of the fiber was not moving, the light pulse in one direction would move from point 1 to point 2, and the pulse in the other direction would move from point 2 to point 1, so the optical path lengths would be equal, and hence the times to traverse this segment would be the same in both directions. However, if the segment is moving with velocity v (noting that a sufficiently short segment for a sufficiently short time is a parallel displacement), the first pulse moves from point 1 to point 2’ during the incremental time dt. In the limit of infinitesimal displacement, the increase in the path length is given by the dot product (v•ds/|ds|)dt, which is also the decrease in path length for the pulse going from point 2 to point 1’. Thus we have

Solving these for the respective time increments and subtracting one from the other, we find that the difference in time for light pulses to traverse this segment in the two directions is

Thus for a belt loop, in which the fiber’s velocity is everywhere parallel to its direction, we have Δt_loop = (2vL)/(c² – v²), as shown previously. To the first order, the total time difference for light pulses to traverse the entire loop in opposite directions is given by the integral

To show how (7) gives the familiar first-order formula for a Sagnac device, suppose the fiber optic loop has a fixed shape and rotates about some arbitrary point as illustrated below.

The velocity v at any point of the loop with coordinates x,y is perpendicular to the radial vector r, so we have v_y/v_x = –x/y. Therefore the integrand of (7) can be written as

Also, since v = ωr, we have

Hence v_x/y = ω, so equation (7) becomes

Recalling that Green’s Theorem implies that the net area enclosed within a closed loop is given by

it follows that

Thus the usual Sagnac formula is given by (7) with the restriction that the loop is rigid and rotating about a fixed point.

Return to MathPages Main Menu