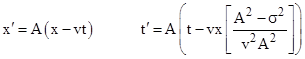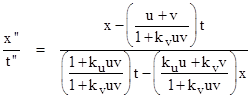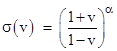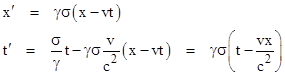Lorentz’s Scale Factor

POINS:         Come, your reason, Jack, your reason.

FALSTAFF:  What, upon compulsion? 'Zounds, an I were at the

                        strappado, or all the racks in the world, I would

                        not tell you on compulsion. Give you a reason on

                        compulsion! If reasons were as plentiful as

                        blackberries, I would give no man a reason upon

                        compulsion, I.

For any material object in any state of motion there exists a system of space and time coordinates with respect to which the object is instantaneously at rest and the isotropic and homogeneous equations of Newton's laws of inertial motion hold good (at least quasi-statically). Such a system is called an inertial rest frame coordinate system of the object.

Let x,t denote inertial rest frame coordinates of one object, and let x′,t′ denote inertial rest frame coordinates of another object moving with a speed v in the positive x direction relative to the x,t coordinates. How are these two coordinate systems related? We can arrange for the origins of the coordinate systems to coincide. Also, since these coordinate systems are defined such that an object in continuous uniform motion with respect to one such system must be in continuous uniform motion with respect to all such systems, and such that inertia is isotropic, it follows that they must be linearly related by the general form

where A,B,C,D are constants for a given value of v. Now, since the second object is stationary at the origin of the x′,t′ coordinates, it's position is always x′ = 0, so the first transformation equation gives 0 = Adx + Bdt, which implies dx/dt = −B/A = v and hence B = −Av. Also, if we solve the two transformation equations for x and t we get

By reciprocity the first object is moving with velocity −v relative to the x′,t′ coordinates, so we have −v = dx'/dt' = B/D, which implies B = −Dv and hence A = D.

At this point we might assert that, by isotropy, the transformation and its inverse must not have different scale factors, so the determinant AD − BC = A² + vAC of the transformation must equal unity. However, in the original (historical) derivations of the Lorentz transformation this was not stipulated. Instead, let σ² denote the value of the determinant (for any given v). Then we have C = (σ² − A²)/(vA). Combining all these facts, a reciprocal transformation from one system of inertial coordinates to another must be of the form

It only remains to determine the values of A and σ (as functions of v), which we can do by fixing the quantity in the square brackets. Letting k denote this quantity for a given v, the transformation can be written in the form

As an aside, we note that the reason for the free scale factor can be seen clearly from another derivation of the Lorentz transformation, which proceeds from the premise that the speed of light is c in terms of every system of inertial coordinates. This implies that if c²t² = x² +y² + z² then c²t′² = x′² + y′² + z′², but we can obviously multiply all the coordinates x′, y′, z′, t′ by a constant σ without affecting the light-speed condition.

Any two inertial coordinate systems must be related by a transformation of the form given above, where v is the mutual speed between them. This implies

Given three systems of inertial coordinates with the mutual speed v between the first two and u between the second two, the transformation from the first to the third is the composition of transformations with parameters k_v and k_u. Letting xʺ,tʺ denote the third system of coordinates, we have by direct substitution

The coefficient of t in the denominator of the right side must be unity, so we have k_u = k_v, and therefore k is a constant for all v, with units of an inverse squared speed. Assuming k is finite, we can by a suitable choice of units set k equal to either −1, 0, or +1. By the reasoning discussed elsewhere we conclude that k = 1, so the above expression reduces to

From this it follows that, letting w denote the mutual speed between the first and third coordinate systems, we have the speed composition relation

We note that this relation does not depend on the value of the scale factor σ(v), so this is consistent with the coordinate transformation

which has the inverse

It follows that σ(−v)σ(v) = 1. It may seem obvious that we should set σ(v) = 1, since this makes the transformations isotropic, but it’s interesting to consider other (non-isotropic) possibilities.

We will stipulate that σ(0) = 1, meaning that, with no relative velocity at all, the two coordinate systems are identical. (Even this might be questioned, because nothing prevents us from defining re-scaled coordinates for the same frame, but this would introduce a singular ambiguity into the descriptions of events.) Furthermore, if the primed coordinates are moving with speed v relative to the unprimed coordinates, and if the double-primed coordinates are moving with speed u relative to the primed coordinates, then by taking the composition of the two transformations we have

where w is the speed given by (1) of the double-primed coordinates relative to the unprimed coordinates, we have σ(w) = σ(v)σ(u). Recalling multiplicative form of the composition rule for velocities, we have

Consequently, a self-consistent transformation is given by setting the scale factor to

where α is an arbitrary constant. Setting α = 0 gives σ(v) = 1, yielding the usual Lorentz transformation, whereas if we set α = 1/2 we get

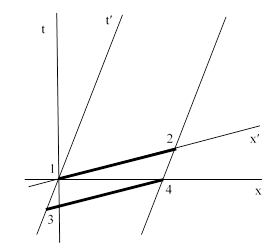
We can choose to define coordinate systems in any way we like (provided they give a one-to-one mapping), but we attach special significance to coordinate systems that conform to physical phenomena. For example, the reason we use Lorentz transformations (α = 0) is because a solid rod that is slowly accelerated to a different state of motion is reconfigured so that it has the same description in terms of the transformed coordinates of the new rest frame. As a result, we have the phenomena of length contraction by a factor of [(1−v)(1+v)]^1/2, which is isotropic, i.e., the contraction is the same for a change in the state of motion of a solid object in any direction. However, if physical phenomena conformed instead to the transformation (2), we would expect that accelerating a solid rod in one direction would result in contraction by a factor of (1–v), and accelerating the rod in the other direction would cause expansion by a factor of (1+v). These factors can be inferred from the figure below.

Letting L₀ denote the length of the rod in terms of the primed coordinates (relative to which it is at rest), the x′ coordinate of event 2 is x′₂ = L₀, and the unprimed coordinates of event 4 are x = L and t = 0, from which we compute that the x′ coordinate of event 4 is x′₄ = L/(1−v). Since x′₂ = x′₄, we have L = L₀(1−v). Replacing with v with –v gives the effect for a rod moving in the opposite direction. In general, the length contraction (or expansion) factor is σ/γ where γ = (1−v²)^−1/2.

Since we don’t observe this kind of anisotropic behavior, we conclude that α must equal zero, or at least be so close to zero that we can’t discern any anisotropy. There are others reasons as well for concluding that α = 0, so one might wonder why anyone would even entertain the possibility that it might be non-zero. However, the early pioneers in the subject, Lorentz and Poincare, both included the σ(v) scale factor in their discussions for a long time, only belatedly adopting the definite value σ(v) = 1, and even then their justifications were somewhat obscure and equivocal.

In his 1904 paper “Electromagnetic Phenomena in a System Moving With Any Velocity Less Than That of Light”, Lorentz developed the final form of the space and time coordinate transformation that bears his name.  First he noted that Maxwell’s equations of electromagnetism are invariant under this transformation, and then he considered the consequences of the postulate that all forces of nature transform in the same way as electromagnetic forces. Finally, he added the postulate that even inertial “forces” transform in the same way, and thereby completed his theorem of corresponding states, the precursor to the modern theory of special relativity. He expresses the transformation between coordinates X,T moving with the system and the “new independent variables” x′,t′ as

where γ = [1 – (v/c)²]^−1/2. Lorentz actually used the symbol β in place of the now more conventional γ, and he used the lower case italic letter l in place of what we call σ. We will refer to this latter quantity as Lorentz’s scale factor. He introduces it here as “another numerical quantity, to be determined further on”, and says that “it is to be considered a function of v, whose value is 1 for v = 0, and which, for small values of v, differs from unity no more than by a quantity of second order”. He carries this scale factor, of undetermined value, throughout almost the entire paper, until finally near the conclusion of the paper he argues that the value is exactly 1, independent of v.

Equations (3) differ in appearance from the familiar form of the Lorentz transformation because the x,t coordinates have already been shifted to “axes moving with the system”, by a Galilean transformation X = x – vt and T = t, where x,t are taken to be stationary inertial coordinates. This implies that X,T are not inertial coordinates, although Lorentz was unclear about this. Making these substitutions, the full transformation from the inertial coordinates x,t to the moving inertial coordinates x′,t′ is

One might expect Lorentz to immediately deduce that σ must equal 1, and omit it from the remaining expressions, but in fact he carries it along as an undetermined quantity in all the expressions in nearly the entire paper. Only toward the end does he deduce its value, and by a somewhat indirect means (and with no mention of isotropy).

He bases his (belated) determination of σ on two different expressions for the so-called longitudinal mass m′ of a particle of rest mass m moving with speed v in the x direction:

In view of the identity

it follows that dσ/dv = 0, so σ is constant, and since σ = 1 at v = 0 we have σ = 1 for all v. Of course, this deduction relies on the two expressions for the longitudinal mass m′, so we need to understand the origins of those expressions.

From the basic transformation equations, and from considerations of electromagnetic forces, he determines that acceleration and force (parallel to the direction of motion) transform according to the relations

This is already somewhat puzzling, for two reasons. First, as discussed in the note on The Inertia of Energy, the transformation a′ = a/γ³ can be derived purely from the velocity composition rule (1), which is independent of the scale factor, so Lorentz’s expression for a′ already implies σ = 1. Second, since Lorentz has already committed to inferring whatever transformation is necessary to fulfill the theorem of corresponding states (i.e., relativity), and since symmetry surely requires that the force of two relatively moving objects on each other along the line of their mutual motions must be equal, his expression for F′ also, by itself, implies σ = 1. But Lorentz doesn’t make use of either of these arguments.

At this point, Lorentz asserts the relations F = ma and F′ = m′a′, and making use of the above expressions for a′ and F′ he finds that the longitudinal mass m′ (i.e., the resistance to acceleration parallel to the direction of motion) is related to the rest mass m by

Lorentz also says that the momentum is given by

and that the transformed force F′ is the derivative of momentum with respect to time, i.e.,

where he has put a′ = dv/dt. (He gives no justification for identifying dv/dt with a′ rather than with a.) Dividing through by a′, he arrives at his second expression for the longitudinal mass

The combination of this and the previous expression for m′ does indeed imply that σ = 1, as explained above, but the reasoning behind these expressions is questionable (which may be why Lorentz delayed for so long in setting σ = 1), and nowhere does Lorentz invoke isotropy, which is seen today as the condition that implies σ = 1.

In 1905, Poincare wrote a detailed exposition of Lorentz’s theory, with some clarifications and corrections (e.g., the current density). Interestingly, Poincare carried the scale factor σ (which he called l, following Lorentz) for most of his discussion, and never actually gives any argument for why it must equal 1. At some point, after σ has appeared in all the expressions as an undetermined parameter (i.e., an “arbitrary constant”), he simply says

To advance further, we need to look for the invariants of the Lorentz group. We know that the substitutions of this group (assuming σ = 1) are linear substitutions that leave unaltered the quadratic form x² + y² + z² – t².

Thus he simply assumes that σ = 1, noting that with this assumption the noted quadratic form is invariant. He gives no justification for this assumption, nor does he comment on Lorentz’s argument, nor does he mention isotropy. (In private correspondence to Lorentz in 1905 Poincare did remark that Lorentz’s argument was inconclusive.) This leaves the impression that Poincare did not see any reason that σ must equal 1 from a physical standpoint, although he obviously recognized the formal appeal of setting σ = 1, even noting that this makes the Lorentz transformations into simple hyperbolic rotations in spacetime.

One of the things that distinguishes Einstein’s 1905 paper from the writings of Lorentz and Poincare is Einstein’s treatment of the scale factor (which Einstein denotes as ϕ). At the very start he sees immediately that applying the coordinate transformations for +v and –v must return us to the original coordinates, so we must have σ(v)σ(−v) = 1. Furthermore, noting that the general transformation of the y coordinates, perpendicular to the x direction of motion, is y′ = σ(v)y, he observes

From reasons of symmetry it is now evident that the length of a given rod moving perpendicularly to its axis, measured in the stationary system, must depend only on the velocity and not on the direction and the sense of the motion... hence σ(v) = σ(−v). It follows from this relation and the one found previously that σ(v) = 1.

Thus he invokes isotropy to argue that the scale factor must equal 1.

Return to MathPages Main Menu