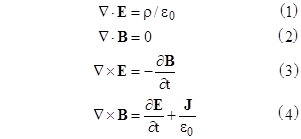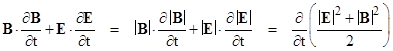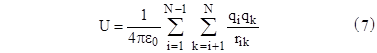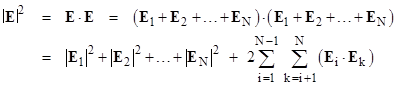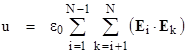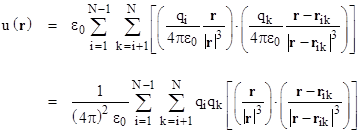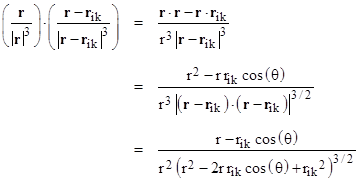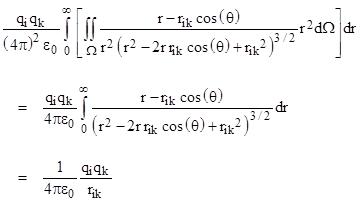Electromagnetic Energy, One Way or Another

The electrostatic energy of the whole field will be the same if we suppose that it resides in every part of the field where electrical force and electrical displacement occur, instead of being confined to the places where free electricity is found. The energy in unit volume is half the product of the electromotive force and the electric displacement, multiplied by the cosine of the angle which these vectors include.

                                        James Clerk Maxwell, Treatise [Sec 631]

The location of the energy associated with electromagnetism can be conceived in at least two seemingly very different ways, corresponding to the distant-action theory of Ampere and Weber, and the field theory of Faraday and Maxwell. If we consider charged particles moving in an empty void and acting on each other at a distance (the Amperean view), we can imagine doing work on a charged particle to move it against the forces exerted by other charged particles. According to this view, the potential energy associated with configurations of charged particles would seem to reside in the particles themselves, on which the work has been performed. This is what Maxwell was referring to when he mentioned the possibility of supposing the energy is confined to the places where free electricity (i.e., charge) is found. As an alternative to this conception, Maxwell suggested that we could suppose the energy permeates the entire region of space (which Maxwell identified with a luminiferous aether) surrounding the charged particles. Remarkably, these two conceptions, seemingly so different, yield exactly the same predictions for all observable phenomena – at least, once the distant-action theory was modified to account for the light-like delay of the retarded potential.

When Maxwell began developing his theory of electromagnetism he tended to conceive of the electric field – albeit somewhat loosely – as a state of stress and strain in a medium surrounding charged particles (“free electricity”). Absent an electric field, he regarded the medium as a manifold of positive and negative elements coinciding with each other (strangely reminiscent of the “Dirac sea”), so the medium was in a relaxed and neutral state. When an electric field was applied, Maxwell thought it “displaced” slightly the positive from the negative components. He regarded even the vacuum of “empty space” as such a dielectric medium, and the electric force E at each point caused a “displacement” D of this medium. In the vacuum, this electric displacement was directly proportional to the applied “force”, i.e., we have D = ε₀E, analogous to how the displacement of a spring is directly proportional to the applied force. The symbol ε₀ denotes the permittivity constant, which is analogous to the spring constant. (In other media, the relationship between the applied electric field and the displacement may be more complicated, and E and D need not be parallel, so it is necessary to take the dot product, which results in the cosine factor mentioned by Maxwell in the above quote.) Just as with a stressed spring, Maxwell naturally thought the stressed medium contained energy, and hence the work done on the medium (in a small unit volume) would be the integral of the force along the displacement. In the vacuum E and D are parallel, so we can deal with just the magnitudes. The electric force is E = D/ε₀, and the integral of this force from a displacement of 0 to D is

So, according to Maxwell, this could be regarded as the energy density of the electric field at any given point. He derived the similar expression (ε₀/2)B² for the energy density of the magnetic field. However, he subsequently downplayed the mechanistic view of the medium and the literal interpretation of the “displacement” vector as an actual spatial displacement. Nevertheless, he found that these expressions for the energy density of the electric and magnetic fields could still be justified, based on more general reasoning from the basic equations governing the electromagnetic field and the interaction with electric charge.

The microscopic Maxwell equations in relativistic SI units (i.e., with ε₀μ₀= 1/c² = 1) can be written in the form

where E and B are the electric and magnetic field vectors, r is the charge density, and J is the current density. If we multiply through equation (3) by -B, and multiply through equation (4) by E, and add the resulting equations, we get

At this point it’s useful to recall the chain rule for the divergence of the cross product of any two vector fields U and V, represented by the identity

Therefore, the expression in square brackets on the left side of equation (5) is simply the divergence of E x B. Also, since any vector and its partial derivative with respect to time point in the same direction, the arguments of the dot products on the right side of equation (5) can be replaced with absolute values, so we have

Combining these results, we can re-write (5) in the form

where S = (E x B)ε₀. We must now interpret each of the terms in this equation. Since E is the force exerted by the electric field at a given point on any incremental charge at that point, and J is the charge density multiplied by the velocity (i.e., J is the current), it follows that the term E∙J is the rate of work being done by the electric field on the moving charge (i.e., the current J) at any given point. Recall that the magnetic field does no work on a charge, as is clear from Lorentz’s force law F = q(E + v x B), which shows the force due to the magnetic field is perpendicular to the velocity. Therefore, the term E∙J at any given point is the entire rate of work being done by the electromagnetic field on any charge at that point. This suggests that equation (6) could be taken to represent an energy principle for electromagnetism. The electromagnetic field clearly performs work on charged particles, so if we want to maintain conservation of energy we need to define some form of potential or field energy that varies in such a way that the total energy is conserved. Furthermore, we recognize the quantity inside the square brackets of (6) as the expressions for the energy density of the electric and magnetic fields that emerge from Maxwell’s early mechanistic conception, as shown by equation (0). Indeed, if we define (ε₀/2)(|E|² + |B|²) as the internal energy density of an electromagnetic field at any given point, and if we define S = (E x B)ε₀ as the flow of electromagnetic energy, then equation (6) implies conservation of energy. The first term on the left side is the divergence of S, which signifies the flow of field energy out of any incremental volume, and the second term (as already mentioned) is the work done by the field on any current that is present. The right hand term is the rate of change of the internal energy.

To show that our identification of (ε₀/2)(|E|² + |B|²) as the field energy density is reasonable and useful, we split it into two parts, and assert that ε₀|E|²/2 and ε₀|B|²/2 are the energy densities of the electric and magnetic fields respectively. Consider first the electric field, and suppose we have N particles with the charges q₁, q₂, …, q_N. By Coulomb’s law of the electrostatic force, the force between the ith and kth particles is

Initially we imagine these particles separated by such great distances from each other that the forces between them are all essentially zero. Conventionally we define the potential energy of this initial configuration as zero, and the work done on or by the particles to bring them (quasi-statically) to some finite separation r_ik is

Therefore, the total potential energy of this static system of charged particles for any given set of pair-wise distances is

This is a palpable measure of energy, derived in terms of the work (force times distance) associated with each possible configuration of charges. This derivation doesn’t mention field energy distributed throughout all of space, it simply associates certain amounts of energy with every pair of charged particles, varying with the work done on or by those particles. However, it turns out that the field energy with density ε₀|E|²/2, integrated over all of space, yields exactly the same expression.

To show this, let the vector fields E₁, E₂, …, E_N denote the contributions of the N charged particles to the electric field at any given location. Thus we have

and therefore

Now, the squared terms in this last expression are independent of the arrangement of the charges. These terms are simply the squared fields of the respective charges. If we were to integrate these terms over the whole volume of space, the result would be infinite, but since these expressions don’t change, we need not include them in our measure of the variable energy of the configuration of charges. This could be seen as a primitive form of re-normalization. Only the changes in energy from one configuration to another are physically significant. The value of ε₀|E|²/2 therefore gives the following expression for the effective internal energy density of the electric field

Recall that if r denotes the position vector of a point P relative to the charge q_i, then the electric field due to q_i at P is

If we let r_ik denote the vector from q_i to q_k, then the vector from q_k to P is r – r_ik, so the energy density is

Now we wish to integrate this, term-by-term, over all r, to get the total energy U of the configuration. The variable factor in the argument of each integration is of the form

where θ is the angle between r and r_ik.  We wish to integrate this over all r from 0 to infinity, and all θ over the spherical angles. Thus the integral for this term is

In the first step the extra factor of r² in the numerator comes from the weighting of the spherical shells of radius r. Integrating over all the solid angles W gives a factor of 4p. Summing this result for all the pair-wise terms, and multiplying by we get exactly the same total energy as given by equation (7). This shows that the change in the integrated value of ε₀|E|²/2 does indeed equal the work done on or by a set of charged particles in changing quasi-statically from one configuration to another. So, in this sense, it seems justifiable to take this expression as the energy density of the electric field E. A similar argument can be given to show that the change in the integral of ε₀|B|²/2 equals the net work involved in imparting velocities to each of the charged particles. Hence we’re justified in regarding (ε₀/2)(|E|² + |B|²) as the total energy density of the electromagnetic field.

As r approaches zero, the volume within the spherical shell of radius of r decreases, so even though the product of the field strengths goes to infinity, the volume integral remains finite. But this is true only for the cross-product terms, for which at most one of the two fields is infinite at any given point. For the squared terms, both factors go to infinity as r approaches zero, and the shrinking volume effect is not sufficient to prevent the integral from being infinite. This is why we had to neglect these terms when evaluating the integral. Our justification for neglecting these infinite contributions was that they don’t change for different configurations, so they don’t contribute to the work. However, they obviously contribute to the absolute value of the “energy density” at each point, so one could question whether those terms should really be included in our definition of the energy density.

The non-uniqueness of energy density functions that comply with the work conditions is one reason it is difficult to identify the absolute background energy level, and to be confident of the localization of field energy in the absolute sense. (The same difficulty faces every field theory, and in fact the ultimate field theory of general relativity doesn’t even support a well-defined localization of energy.) Notice that the contributions of the squared terms are not just some constant value, they vary continuously throughout the field, and are finite at all points except the charge locations, so they contribute in a non-trivial way to the shape and content of the field energy that we claim is residing at each point in space – even though these contributions are disregarded in our assessment of the work.

Some approaches to electrodynamics have tried to avoid the infinite self-energy problem by assuming that elementary charged particles do not act on themselves – a point of view that seems more consistent with the distant action model rather than the field model. Of course, the prospect of infinite energy in the field model arises only if we assume our charged particles are point-like, i.e., that they have zero radius. In the early years of the development of electrodynamics it was usually assumed that electrons had some finite radius, which avoided the infinite energy problem but which led to other problems, such as the need to explain how the distributed charged substance was held together, i.e., it was necessary to postulate some other forces of coherence to counter-act the mutual repulsion of the parts of an electron.

If we accept the identification of (ε₀/2)(|E|² + |B|²) as the total energy density of the electromagnetic field, it follows that the vector field S = (E x B)ε₀ represents the flow of electromagnetic energy. This is called Poynting’s vector, named after the English physicist John Henry Poynting who first described it in 1884. It also implies the corresponding flow of momentum, and is very important for a clear understanding of relativistic electrodynamics. Despite its importance, the Poynting vector is (as already noted) not uniquely determined. In fact, it can be augmented by any divergence-free vector field without affecting any of the measurable phenomena. For this reason, and also because some of the implications of Poynting’s theorem are counter-intuitive, many physicists have been hesitant about accepting it at face value. Even Maxwell himself seemed slightly equivocal when we wrote in 1873 that “the electrostatic energy of the whole field will be the same if we suppose that it resides in every part of the field where electrical force and electrical displacement occur”. As late as 1909 (and in the second edition published in 1915) Lorentz wrote

The flow of energy can, in my opinion, never have quite the same distinct meaning as a flow of material particles… It might even be questioned whether, in electromagnetic phenomena, the transfer of energy really takes place in the way indicated by Poynting’s law, whether, for example, the heat developed in the wire of an incandescent lamp is really due to energy which it receives from the surrounding medium, as the theorem teaches us, and not to a flow of energy along the wire itself. In fact, all depends upon the hypotheses which we make concerning the internal forces in the system, and it may very well be that a change in these hypotheses would materially alter our ideas about the path along which the energy is carried from one part of the system to another. It must be observed however that there is no longer room for any doubt, so soon as we admit that the phenomena going on in some part of the ether are entirely determined by the electric and magnetic force existing in that part.  Therefore, if all depends on the electric and magnetic force, there must also be one near the surface of a wire carrying a current, because here, as well as in a beam of light, the two forces exist at the same time and are perpendicular to each other.

It’s interesting that while having a clear and lucid grasp of the accepted explanations, Lorentz always remained open to the possibility of other forces, not presently identified or recognized, that might significantly alter our ideas. He took the same attitude toward the principle of relativity, conceding that if all physical phenomena were entirely relativistic, then his conceptions of the ether and absolute time lose their meaning, but he nevertheless persisted in thinking it was best “not to assume at starting that it can never make any difference whether a body moves through the ether or not”. Thus he argued that we should “measure distances and lengths of time by means of rods and clocks having a fixed position relatively to the ether”, because he expected some presently unknown forces or effects violating Lorentz invariance might eventually be discovered. However, his position was somewhat incoherent, since he also admitted that, until such a violation of Lorentz invariance is found, there is (by definition) no way of determining the putative rest frame of the ether, so his advice can’t actually be followed.

Incidentally, the example of the energy flow into a conducting wire cited by Lorentz had been discussed previously by Poynting himself, and also by the British electrician Oliver Heaviside in 1887. Heaviside noted that the electric field vector inside the wire is parallel to the wire, so the energy flux vector inside the wire points directly inward. Outside the wire, the electric field vector is nearly (but not quite) perpendicular to the wire, so the energy flux vector is nearly parallel to the wire, although it does converge slightly into the wire, consistent with Lorentz’s statement that the heat enters the wire from the surrounding field. This shows that, assuming we accept the standard form and interpretation of Poynting’s vector, the flow of energy in the direction of a conducting wire is entirely in the region outside the wire itself.

Return to MathPages Main Menu