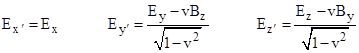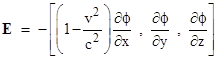Aberration of Forces and Waves

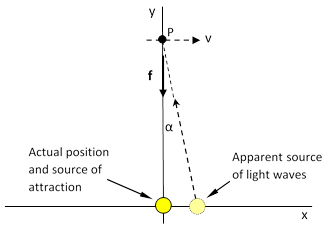
Consider an illuminated charged sphere resting at the origin of a system x,y,z of inertial coordinates, and a small test particle moving with speed v in the positive x direction at a fixed y coordinate as shown below.

If the distance between the two objects is sufficiently great, the light (electromagnetic waves) emanating from the sphere will consist of essentially planar horizontal waves when it reaches the test particle. Since the particle is moving tangentially with speed v, the angle of the incoming light will be affected by aberration, such that the apparent source of the light (from the point of view of the test particle as it crosses the y axis) is at an angle α = arcsin(v/c) ahead of the actual position of the sphere. However, the direction of the electrical force exerted by the sphere on the test particle points directly toward the actual position of the sphere. Thus, the incoming electromagnetic waves from the sphere experience aberration, but the electromagnetic force of attraction to the sphere does not. This sometimes misleads people into thinking that the force somehow propagates instantaneously (to account for the absence of aberration).

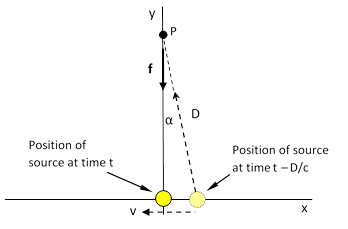
Of course we can just as well consider the test particle to be at rest, and the charged sphere to be moving in the negative x direction with speed v. From this point of view, if D denotes the distance from the sphere to the particle, then at any time t the particle “sees” the sphere at the location it occupied at a time t − D/c, because D/c is how long it take for light to travel the distance D. This is illustrated in the figure below.

Just as before, the light arrives at the (stationary) particle P from a direction differing from the true current direction of the source at time t by the angle α. Also, since we have simply changed coordinate systems, which can have no effect on any physical attributes, we know the electromagnetic force on the particle at the time t points directly toward the sphere’s actual (not apparent) position at that time.

The absence of aberration in the direction of the electromagnetic force does not indicate that the force propagates infinitely fast. (In fact, the concept of a “moving force” is not even well defined.) The force on a test particle at any given instant is due to the electromagnetic field in the immediate vicinity of the particle at that instant. In general the field at any given place and time consists of contributions from multiple sources at a variety of distances. The number of sources and their distances matter only insofar as they determine the electromagnetic field. The field of the charged sphere with respect to the rest frame of the sphere is an electro-static configuration (no magnetic field) with spherical symmetry centered on the source. A uniformly moving charged test particle in this field is subjected to a force proportional to (and therefore pointing in the same direction as) the electric field vector at its present location, so the force obviously points directly towards the source at all times. On the other hand, in terms of the rest frame of the test particle the charged sphere is in uniform motion and the electromagnetic field has both electric and magnetic components. However, since the test particle is at rest with respect to these coordinates, it does not experience any magnetic force, so again the force on the particle is proportional to the electric field vector. To determine the direction of this force we need to know how the components of the electric field transform from one system of inertial coordinates to another. As explained in Force Laws and Maxwell’s Equations, if E_x, E_y, and E_z are the components of the electric field at a given point with respect to the x,y,z,t coordinates, then the components with respect to a similarly oriented system of inertial coordinates x′,y′,z′,t′ moving with speed v in the positive x direction are

Of course we also have

In the unprimed coordinates (the rest frame of the charged sphere) we know the electric field components at the location of the test particle point directly toward the origin, which means E_x, E_y, and E_z are proportional to the coordinates x, y, and z of the test particle. Also, since the magnetic field is zero with respect to the unprimed coordinates, and since the origins of the two coordinate systems coincide at t = 0, we have

Similarly it follows that E_x′/E_z′ = x′/z′ and E_y′/E_z′ = y′/z′, confirming that the electric field vector at every location points directly toward the instantaneous source with respect to the rest frame of the test particle (relative to which the source is moving with a speed v). Thus the absence of “force aberration” for objects in fully developed inertial motion is an immediate consequence of Lorentz covariance.

The qualifier “fully developed” is necessary, because every object is instantaneously at rest with respect to some inertial frame, but the object’s field in its current rest frame is spherical and satisfies the steady-state relations only out to a distance D = cΔt where Δt is the length of time the object has been unaccelerated. This highlights the fact that although the field of an object exists and acts at a distance from the object, changes in the field propagate at the finite speed c. When an object changes its state of motion, the field must change accordingly, and these changes propagate outward from the source at the speed c. In the far field these changes propagate in the form of waves.

One thing that sometimes puzzles people about the lack of force aberration is that they tend to regard the electric field as the gradient of a potential, and they know the equi-potential surfaces for a uniformly moving charged particle are contracted in the direction of motion so they form ellipsoids instead of spheres, and clearly the spatial gradient of this potential does not point towards the center (except for lines parallel or perpendicular to the axis of motion). The explanation is that the electric field vector equals the spatial gradient of the potential field only if the field is stationary, i.e., unchanging with time. If the field is changing with time, the full expression for the electric field must include an additional term to account for this, i.e., we have

where A signifies the vector potential of the electromagnetic field. The second term on the right hand side represents the effect of the changing potential with time. Using the Lorentz gage

the field equations for the electromagnetic potentials are

It follows that, if v is constant (and has been for a sufficiently long time), and we are given a solution ϕ(x,y,z,t) for the scalar potential, we can multiply this solution by v/c to give a solution of the vector potential

Therefore, under these conditions, the time-dependent term in the last equation for E can be written as

Now, by definition, the total derivative of ϕ along any incremental path dx,dy,dz,dt is

Dividing by dt and solving for the partial of ϕ with respect to t gives

Taking dx/dt etc. as the components of the sphere’s velocity v, the total derivative dϕ/dt represents the change in ϕ with time along a co-moving worldline, and since the (fully developed) field is stationary with respect to the rest frame of the sphere, we have dϕ/dt = 0. Therefore the partial of f with respect to t equals the negative of the dot product of the spatial gradient of ϕ with the velocity v, so the previous expression for the time-dependent electric field is

We are considering the case when the sphere’s motion is in the positive x direction, so we have v = (v,0,0) and the above expression becomes

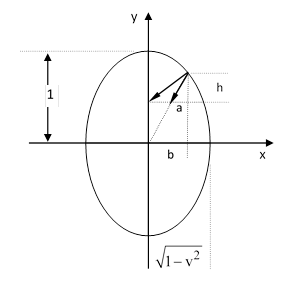
A surface of constant ϕ is a stationary sphere in the rest frame of the source, so it transforms to an ellipsoid due to contraction in the direction of motion as shown below.

The equation of this cross-section is

where we have chosen units so that c = 1. Taking the differential of both sides gives

The slope of the normal to the ellipse at the point (x,y) is the negative reciprocal of dy/dx,, which is

According to our expression for E(r,t) we begin with the gradient of ϕ and then reduce the x component by the factor (1 − v²), where we still have c = 1. Thus we have

This confirms that the electromagnetic force exerted by the field of the moving charged sphere on the test particle at time t is directed toward the position of the sphere at the same time t. This is a natural consequence of Lorentz covariance, and does not imply any instantaneous transfer of energy or information.

It’s true that, in quantum theory, the electromagnetic force can be considered to be mediated by photons, but these are virtual photons, which are actually just analytical components of the field. In effect these virtual photons form a cloud around the source particle, and they “exist” only within the uncertainty envelope. An electromagnetic interaction between two electrons, for example, is modeled as an exchange of photons between the overlapping fields of two particles. It is not represented by a photon traversing from one particle to the other. Virtual photons don’t even possess definite trajectories through space and time. They are conceptual entities arising in the quantization of the electromagnetic field.

Another point that sometimes puzzles people is why an equi-potential sphere transforms to an ellipsoid under a Lorentz transformation, whereas a spherical wave of light transforms to a spherical wave under the same transformation. The reason is that an equi-potential sphere is stationary, whereas a wave of light is expanding, as illustrated below.

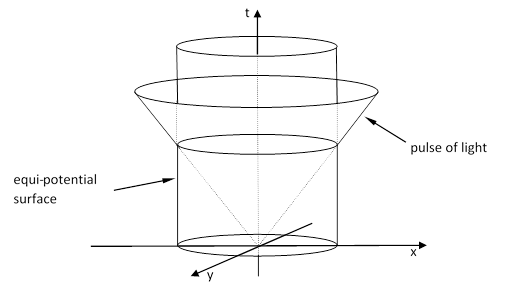
A side view showing the intersections of these two surfaces with two difference planes of simultaneity is shown below.

The source of the light pulse and the potential field moves along the t axis. With respect to the x,t coordinate system the expanding spherical shell of light coincides with an equi-potential sphere at the time t = k with the diameter AB. However, with respect to the x′,t′ coordinate system the left-most point of the expanding sphere of light just touches the left-most point of the equi-potential sphere at the point A and time t′ = k′. At this time the right-most point of the light sphere is at C, whereas the right-most point of the equi-potential surface is at D. The “center” of the expanding light sphere (with respect to the primed coordinates) moves along the t’ axis, whereas the center of the potential still moves along the t axis. This illustrates why the coincidence of the light and the equi-potential spheres (at a particular instant) with respect to one frame of reference does not imply that they ever coincide with respect to another frame of reference. The wavefront of the light pulse is always spherical with respect to both systems of inertial coordinates, whereas the equi-potential surfaces are spherical only with respect to the rest frame of the source.

Return to MathPages Main Menu