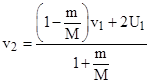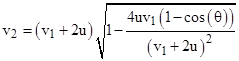Gravitational Slingshot

Interplanetary space probes often make use of the "gravitational slingshot" effect to propel them to high velocities. For example, Voyager 2 performed a close flyby of Saturn on the 27th of August in 1981, which had the effect of slinging it toward its flyby of Uranus on the 30th of January in 1986. Since gravity is a conservative force, it may seem strange that an object can achieve a net gain in speed due to a close encounter with a large gravitating mass. We might imagine that the speed it gains while approaching the planet would be lost when receding from the planet. However, this is not the case, as we can see from simple consideration of the kinetic energy and momentum, which shows how a planet can transfer kinetic energy to the spacecraft.

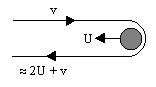
An extreme form of the maneuver would be to approach a planet head-on at a speed v while the planet is moving directly toward us at a speed U (both speeds defined relative to the "fixed" Solar frame). If we aim just right we can loop around behind the planet in an extremely eccentric hyperbolic orbit, making a virtual 180-degree turn, as illustrated below.

The net effect is almost as if we "bounced" off the front of the planet. From the planet's perspective we approached at the speed U+v, and therefore we will also recede at the speed U+v relative to the planet, but the planet is still moving at (virtually) the speed U, so we will be moving at speed 2U+v. This is just like a very small billiard ball bouncing off a very large one.

To be a little more precise, conservation of kinetic energy and momentum before and after the interaction requires

where subscripts 1 and 2 denote before and after, respectively. We eliminate U₂ and solve for v₂ to give the result

Since m/M is virtually zero (the probe has negligible mass compared with the planet), this reduces to our previous estimate of v₂ = v₁ + 2U₁.

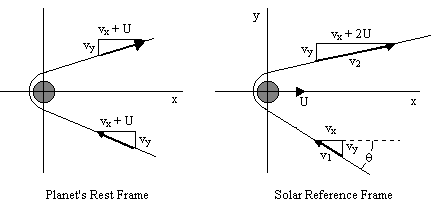
Of course, most planetary fly-bys are not simple head-on reversals, but the same principles apply for any angle of interaction. Let's take the planet's direction of motion as the x axis, and the perpendicular direction (in the orbital plane) as the y axis. The probe is initially moving with a speed v relative to the solar reference frame, in a direction approaching the oncoming planet at an angle θ. Two views of this are shown below, one with respect to the planet's rest frame, and the other with respect to the solar reference frame.

By drawing a simple parallogram of speeds for the probe and planet intersecting at an arbitrary angle θ, and assuming we arrange for a hyperbolic orbit symmetrical about the x axis (with respect to the planet's rest frame), the probe's initial velocity vector with respect to the Sun's rest frame is

and its final velocity vector is

Thus its initial magnitude is v₁, and its final magnitude is

For example, suppose the initial speeds of the probe and the planet happen to be exactly the same (i.e., v₁ = U). In this case the above relation reduces to

which confirms that when θ = 0 we have v₂ = 3v₁, which is our head-on reversal case. On the other hand, when θ = π we have v₂ = v₁, which stands to reason, because in this case the probe and planet are going in the same direction at the same speed. For a more realistic case, we can have the probe approach nearly perpendicular to the planet's path (i.e., θ = π/2) and swing just behind it. In that case the probe gets deflected in the direction of the planet's travel, at an angle given by the above formulas, and it's final speed is the square root of 5 (i.e., about 2.23) times its original speed.

If the planets were point particles, then according to classical physics it would be theoretically possible (in some rather contrived solar systems) for an object to acquire infinite speed in finite time by looping repeatedly around a set of planets. Of course, since actual planets are not point-like, the external gravitational field of an ordinary planet would not be strong enough to "grab" the spaceship once it was traveling above a certain speed. The limit is how fast we can loop around a planet without dipping into its atmosphere too deeply (let alone crashing into it). Some NASA missions have repeatedly skimmed the upper atmospheres of Venus and the Earth in their maneuvers (cross- pollinating the environments?).

Conceivably, if we (or someone else) ever found a star system consisting of multiple black holes orbiting each other, it might be possible to apply this scheme to achieve relativistic speeds, by looping around from one to the other. In this situation the achievable speed limit would depend on how close a spaceship could pass without being destroyed by tidal forces. Still, if the black holes were large enough, the tidal forces even at the event horizon would be tolerable, although it probably wouldn't be possible to have a controllable hyperbolic orbit pass closer than, say 3m. Also, stopping the vehicle at the destination would be difficult.

Return to MathPages Main Menu